When valuing a new venture by a company, it is necessary to use an appropriate discount rate. However, since corporations can be structured very differently, it is important to reflect that in the respective costs of capital. Let's say there are two similar companies in the same industry. Company A is financed 90% by equity (that is, stock) and 10% by debt (long term corporate bonds). Company B is financed by 25% equity, and 75% debt. These two companies would have to be valued according to their respective risk levels and required returns.
One common way to determine the cost of capital is to use the Weighted Average Cost of Capital, or WACC.
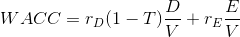
In this formula, V is equal to the value of the firm, or Debt (D) plus Equity (E) Example:
AKL corporation is currently financed with $1,000,000 of 7% bonds, and $2,000,000 of common stock. The stock has a beta of 1.5, and the risk free rate is 4%, and the market risk premium is 3.5%. The marginal tax rate for a corporation of AKL's size is 35%. What is AKL's WACC?
The first thing we must do in this problem is determine the required rate on equity (Re) for AKL. We can plug the Beta given and the risk free rate into the CAPM as follows:
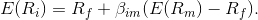
where:
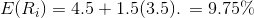
Now, we have all of the necessary information to solve for WACC:
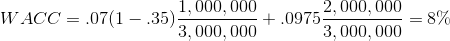
- 3445 reads






