When building a portfolio, it is important not only to consider the individual securities in a portfolio, but also how they interact with one another. For example, consider a portfolio that contains two stocks, XYZ and ABC.
The following formula finds the variance of a portfolio using the correlation (rho) between two stocks in a portfolio:
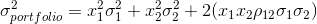
Where x represents the weight of each security in the portfolio.
So, let us suppose the following information:
| XYZ | ABC | |
| Price | $50 | $60 |
| Shares | 20 | 15 |
| Variance | 0.02 | 0.04 |
| Std. Dev. | 0.1414 | 0.2 |
And the correlation between the two is 0.25
Find the variance of this portfolio.
The first thing we must do is determine the relative weights of each stock. We see that we have $1000 in XYZ, and 900 in ABC. Therefore x1=0.53 and x2=0.47. The other relevant information is listed, so we simply plug it into the equation:
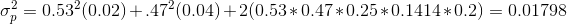
Now let us say we were trying to find the Beta of the XYZ company, and we know that the market has a variance of 0.04. The following formula will allow us to do so:
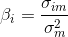
First, we will need to find the covariance (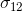 ) between the market and XYZ.
For that, we will use the following formula:
) between the market and XYZ.
For that, we will use the following formula:

For this exercise we will need to know the correlation between the market and XYZ, which we will say is 0.75. Therefore:
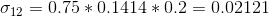
Applying our covariance of 0.02121 to the formula for beta, we find that:
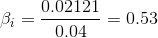
- 3053 reads






