Electrical communications channels are either wireline or wireless channels. Wireline channels physically connect transmitter to receiver with a "wire" which could be a twisted pair, coaxial cable or optic fiber. Consequently, wireline channels are more private and much less prone to interference. Simple wireline channels connect a single transmitter to a single receiver: a point-to-point connection as with the telephone. Listening in on a conversation requires that the wire be tapped and the voltage measured. Some wireline channels operate in broadcast modes: one or more transmitter is connected to several receivers. One simple example of this situation is cable television. Computer networks can be found that operate in point-to-point or in broadcast modes. Wireless channels are much more public, with a transmitter's antenna radiating a signal that can be received by any antenna sufficiently close enough. In contrast to wireline channels where the receiver takes in only the transmitter's signal, the receiver's antenna will react to electromagnetic radiation coming from any source. This feature has two faces: The smiley face says that a receiver can take in transmissions from any source, letting receiver electronics select wanted signals and disregarding others, thereby allowing portable transmission and reception, while the frowny face says that interference and noise are much more prevalent than in wireline situations. A noisier channel subject to interference compromises the flexibility of wireless communication.
NOTE: You will hear the term tetherless networking applied to completely wireless computer
networks.
Maxwell's equations neatly summarize the physics of all electromagnetic phenomena, including cir cuits, radio, and optic fiber transmission.
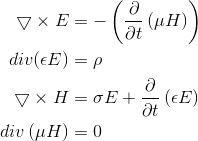
(6.1)
where E is the electric field, H the magnetic field, ϵ dielectric permittivity, µ magnetic permeability, σ electrical conductivity, and ρ is the charge density. Kirchof's Laws represent special cases of these equations for circuits. We are not going to solve Maxwell's equations here; do bear in mind that a fundamental understanding of communications channels ultimately depends on fluency with Maxwell's equations. Perhaps the most important aspect of them is that they are linear with respect to the electrical and magnetic fields. Thus, the fields (and therefore the voltages and currents) resulting from two or more sources will add.
- 瀏覽次數:7349






