We have two producers and two goods: Amanda and Zoe produce vegetables (V) and/or fish (F). Their production capabilities are defined in Table 1.1 and in Figure 1.1, where the quantity of V appears on the vertical axis and the quantity of F on the horizontal axis. Zoe and Amanda each have 36-hour weeks and they devote that time to producing the two goods. But their efficiencies differ: Amanda requires two hours to produce a unit of V and three hours for a unit of F. As a consequence, if she devotes all of her time to V she can produce 18 units, or if she devotes all of her time to F she can produce 12 units. Or, she could share her time between the two.
|
Hours/ Fish |
Hours/ Vegetable |
Fish production |
Vegetable production |
|
|
Amanda |
3 |
2 |
12 |
18 |
|
Zoe |
2 |
4 |
18 |
9 |
Each producer has a time allocation of 36 hours. By allocating total time to one activity, Amanda can produce 12F or 18V, Zoe can produce 18F or 9V. By splitting their time each person can also produce a combination of the two.
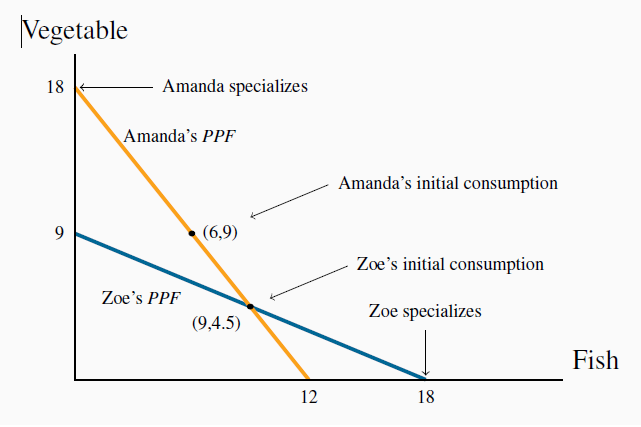
In Figure 1.1 Amanda’s capacity is represented by the line that meets the vertical axis at 18 and the horizontal axis at 12. The vertical point indicates that she can produce 18 units of V if she produces zero units of F – keep in mind that where V has a value of 18, Amanda has no time left for fish production. Likewise, if she devotes all of her time to fish she can produce 12 units, since each unit requires 3 of her 36 hours. The point F = 12 is thus another possibility for her. In addition to these two possibilities, which we can term ’specialization’, she could allocate her time to producing some of each good. For example, by dividing her 36 hours equally she could produce 6 units of F and 9 units of V. A little computation will quickly convince us that different allocations of her time will lead to combinations of the two goods that lie along a straight line joining the specialization points. We will call this straight line Amanda’s production possibility frontier (PPF): it is the combination of goods she can produce while using all of her resources – time. She could not produce combinations of goods represented by points beyond this line (to the top right). She could indeed produce combinations below it (lower left) – for example a combination of 4 units of V and 4 units of F; but such points would not require all of her time. The (4,4) combination would require just 20 hours. In sum, points beyond this line are not feasible, and points within it do not require all of her time resources.
 The production possibility frontier (PPF)
defines the combination of goods that can be produced using all of the resources available.
The production possibility frontier (PPF)
defines the combination of goods that can be produced using all of the resources available.
Having developed Amanda’s PPF, it is straightforward to develop a corresponding set of possibilities for Zoe. If she requires 4 hours to produce a unit of V and 2 hours to produce a unit of F, then her 36 hours will enable her to specialize in 9 units of V or 18 units of F; or she could produce a combination represented by the straight line that joins these two specialty extremes.
Consider now what we term the opportunity costs for each person. If Amanda, from a starting point of 18 V and zero F, wishes to produce some F and less V, she must sacrifice 1.5 units of V for each unit of F she decides to produce. This is because F requires 50% more hours than V. Her trade-off is 1.5:1.0, or equivalently 3:2. In the graphic, for every 3 units of V she does not produce she can produce 2 units of F, reflecting the hours she must devote to each. Yet another way to see this is to recognize that if she stopped producing the 18 units of V entirely, she could produce 12 units of F; and the ratio 18:12 is again 3:2. This then is her opportunity cost: the cost of an additional two units of F is that 3 units of V must be ’sacrificed’.
Zoe’s opportunity cost, by the same reasoning, is 1:2 – 1 unit of V for 2 units of F.
So we have established two things about Amanda and Zoe’s production possibilities. First, if Amanda specializes in V she can produce more than Zoe, just as Zoe can produce more than Amanda if Zoe specializes in F. Second, their opportunity costs are different: Amanda must sacrifice more V than Zoe in producing one more unit of F.
To illustrate the gains from specialization and trade, let us initially suppose that Amanda and Zoe are completely self-sufficient (they consume exactly what they produce), and they each divide their production time equally between the two goods. Hence, Amanda produces and consumes 6F and 9V, whereas Zoe’s combination is 9F and 4.5V. These combinations must lie on their respective PPFs and are illustrated in Figure 1.1.
Upon realizing that they are not equally efficient in producing the two goods, they decide to specialize completely in producing just the single good where they are most efficient. Amanda specializes in V and Zoe in F. Right away we notice that this allocation of time will realize 18V and 18F, which is more than the combined amounts they produce and consume when not specializing – 15F and 13.5V. Logic dictates that each should be able to consume more following specialization. What they must do however, is negotiate a rate at which to exchange V for F. Since Amanda’s opportunity cost is 3:2 and Zoe’s is 1:2, perhaps they agree to exchange V for F at an intermediate rate of 2:2 (or 1:1, which is the same). With Amanda specializing in V and Zoe in F they now trade one unit of V for one unit of F. Consider Figure 1.2.
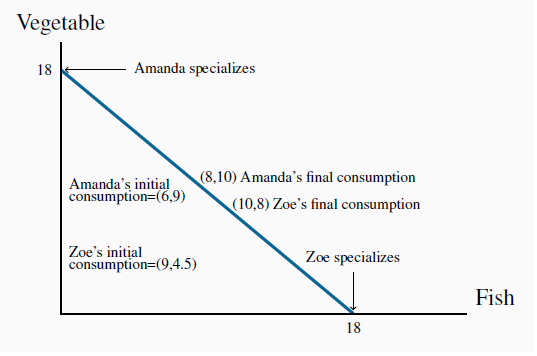
If Amanda can trade at a rate of 1:1 her consumption opportunities have improved dramatically: if she were to trade away all of her 18V, she would get 18 fish in return, whereas when consuming what she produced, she was limited to 12 fish. Suppose she wants to consume both V and F and she offers Zoe 8V. Clearly she will get 8F in return, and she will consume (8F+10V) – more than she consumed prior to specializing.
By the same reasoning, after specializing in producing 18 fish, Zoe trades away 8F and receives 8V from Amanda in return. Therefore Zoe consumes (10F+8V). The result is that they are now each consuming more than in the initial allocation. Specialization and trade have increased their consumption. 1
- 瀏覽次數:3478






