Calculating the Sample Size n

Available under Creative Commons-ShareAlike 4.0 International License. Download for free at http://cnx.org/contents/733d1554-5d75-4798-9e54-7dcdc1ee5690@5.40

If researchers desire a specific margin of error, then they can use the error bound formula to calculate the required sample size. The error bound formula for a population proportion is

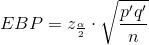
$EBP=z_{\frac{\alpha}{2}}\cdot \sqrt{\frac{p'q'}{n}}$
Solving for n gives you an equation for the sample size.
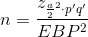
$n=\frac{z_{\frac{a}{2}^{2}\cdot p'q'}}{EBP^{2}}$

Example 4.10

Suppose a mobile phone company wants to determine the current percentage of customers aged 50+ that use text messaging on their cell phone. How many customers aged 50+ should the company survey in order to be 90% confident that the estimated (sample) proportion is within 3 percentage points of the true population proportion of customers aged 50+ that use text messaging on their cell phone.

Solution
From the problem, we know that EBP=0.03 (3% 0.03) and
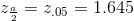$z_{\frac{a}{2}}=z_{.05} =1.645$ because the confidence level is 90%

However, in order to find n , we need to know the estimated (sample) proportion p'. Remember that q' = 1-p'. But, we do not know p' yet. Since we multiply p' and q' together, we make them both equal to 0.5 because p'q' = (.5)(.5) = .25 results in the largest possible product. (Try other products: (.6)(.4) = .24; (.3)(.7) = .21; (.2)(.8) = .16 and so on). The largest possible product gives us the largest n. This gives us a large enough sample so that we can be 90% confident that we are within 3 percentage points of the true population proportion. To calculate the sample size n, use the formula and make the substitutions.
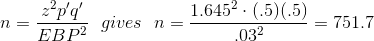
$n=\frac{z^{2} p'q'}{EBP^{2}}\ \ gives\ \ n=\frac{1.645^{2}\cdot (.5)(.5)}{.03^{2}}=751.7$
Round the answer to the next higher value. The sample size should be 752 cell phone customers aged 50+ in order to be 90% confident that the estimated (sample) proportion is within 3 percentage points of the true population proportion of all customers aged 50+ that use text messaging on their cell phone.

**With contributions from Roberta Bloom.

2245 reads

You are here

Calculating the Sample Size n