Capacitor

Available under Creative Commons-ShareAlike 4.0 International License.

Figure 3.3 Capacitor.

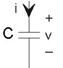
The capacitor stores charge and the relationship between the charge stored and the resultant voltage is q = Cv. The constant of proportionality, the capacitance, has units of farads (F), and is named for the English experimental physicist Michae l Faraday. As current is the rate of change of charge, the v-i relation can be expressed in differential or integral form.

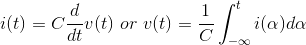
$i(t)=C\frac{d}{dt}v(t)\ or\ v(t)=\frac{1}{C}\int_{-\infty }^{t}i(\alpha)d\alpha$

If the voltage across a capacitor is constant, then the current flowing into it equals zero. In this situation, the capacitor is equivalent to an open circuit. The power consumed/produced by a voltage applied to a capacitor depends on the product of the voltage and its derivative.

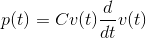
$p(t)=Cv(t)\frac{d}{dt}v(t)$

This result means that a capacitor's total energy expenditure up to time t is concisely given by

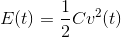
$E(t)=\frac{1}{2}Cv^2(t)$

This expression presumes the fundamental assumption of circuit theory: all voltages and currents in any circuit were zero in the far distant past (t = −∞).

瀏覽次數：1484

您在這裡

Capacitor