We can find voltages and currents in simple circuits containing resistors and voltage or current sources. We should examine whether these circuits variables obey the Conservation of Power principle: since a circuit is a closed system, it should not dissipate or create energy. For the moment, our approach is to investigate first a resistor circuit's power consumption/creation. Later, we will prove that because of KVL and KCL all circuits conserve power.
As defined previously, the instantaneous power consumed/created by every circuit element equals the product of its voltage and current. The total power consumed/created by a circuit equals the sum of each element's power.
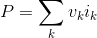
Recall that each element's current and voltage must obey the convention that positive current is defined to enter the positive-voltage terminal. With this convention, a positive value of vkik corresponds to consumed power, a negative value to created power. Because the total power in a circuit must be zero (P =0), some circuit elements must create power while others consume it.
Consider the simple series circuit should in Electric Circuits and Interconnection Laws. In performing our calculations, we defined the current iout to flow through the positive-voltage terminals of both resistors and
found it to equal 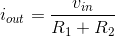 . The voltage across the resistor
R2 is the output voltage and we found it to equal
. The voltage across the resistor
R2 is the output voltage and we found it to equal 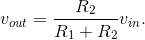
Consequently, calculating the power for this resistor yields
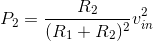
Consequently, this resistor dissipates power because P2 is positive. This result should not be surprising since we showed (p. 41) that the power consumed by any resistor equals either of the following.
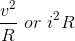
Since resistors are positive-valued, resistors always dissipate power. But where does a resistor's power go? By Conservation of Power, the dissipated power must be absorbed somewhere. The answer is not directly predicted by circuit theory, but is by physics. Current fowing through a resistor makes it hot; its power is dissipated by heat.

The quantity ρ is known as the resistivity and presents the resistance of a unit-length, unit cross-sectional area material constituting the wire. Resistivity has units of ohm-meters. Most materials have a positive value for ρ, which means the longer the wire, the greater the resistance and thus the power dissipated. The thicker the wire, the smaller the resistance. Superconductors have zero resistivity and hence do not dissipate power. If a room-temperature superconductor could be found, electric power could be sent through power lines without loss!
Exercise 3.5.1
Calculate the power consumed/created by the resistor R1 in our simple circuit example. We conclude that both resistors in our example circuit consume power, which points to the voltage source
as the producer of power. The current flowing into the source's positive terminal is −iout . Consequently, the power calculation for the source yields
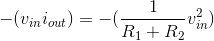
We conclude that the source provides the power consumed by the resistors, no more, no less.
Exercise 3.5.2
Confirm that the source produces exactly the total power consumed by both resistors.
This result is quite general: sources produce power and the circuit elements, especially resistors, consume it. But where do sources get their power? Again, circuit theory does not model how
sources are constructed, but the theory decrees that all sources must be provided energy to work.
- 6292 reads






