The independent-samples t test is
used to compare the means of two separate samples (M1and M2). The two samples might have been tested under different conditions in a between-subjects
experiment, or they could be preexisting groups in a correlational design (e.g., women and men, extroverts and introverts). The null hypothesis is that the means of the two populations are the
same: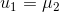 
. The alternative hypothesis is that they are not the
same: µ1 ≠ µ2. Again, the test can be one-tailed if the researcher has good reason to expect the difference goes in a particular direction.
The t statistic here is a bit more complicated because it must take into account two sample means, two standard deviations, and two sample sizes. The formula is as follows:
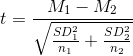
Notice that this formula includes squared standard deviations (the variances) that appear inside the square root symbol. Also, lowercase n1 and n2 refer to the sample sizes in the two groups or condition (as opposed to capital N, which generally refers to the total sample size). The only additional thing to know here is that there are N− 2 degrees of freedom for the independent-samples t test.
- 1462 reads
