Available under Creative Commons-ShareAlike 4.0 International License.
Suppose we have a list of numbers 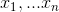 , and we wish to find
some number
, and we wish to find
some number 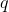 that is as close as possible to as many of
the
that is as close as possible to as many of
the 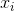 as possible. To make this a mathematically precise
goal, we need to define some numerical measure of this closeness. Suppose we let
as possible. To make this a mathematically precise
goal, we need to define some numerical measure of this closeness. Suppose we let 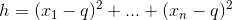 , which can also be notated using
, which can also be notated using 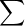 , uppercase Greek sigma, as
, uppercase Greek sigma, as 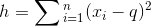 . Then minimizing
. Then minimizing 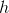 can be used as a definition of optimal closeness. (Why would we not want to use
can be used as a definition of optimal closeness. (Why would we not want to use 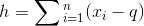 ?) Prove that the value of
?) Prove that the value of 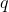 that
minimizes
that
minimizes 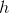 is the average of the
is the average of the 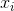 .
.
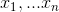 , and we wish to find
some number
, and we wish to find
some number 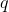 that is as close as possible to as many of
the
that is as close as possible to as many of
the 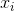 as possible. To make this a mathematically precise
goal, we need to define some numerical measure of this closeness. Suppose we let
as possible. To make this a mathematically precise
goal, we need to define some numerical measure of this closeness. Suppose we let 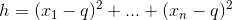 , which can also be notated using
, which can also be notated using 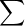 , uppercase Greek sigma, as
, uppercase Greek sigma, as 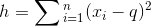 . Then minimizing
. Then minimizing 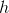 can be used as a definition of optimal closeness. (Why would we not want to use
can be used as a definition of optimal closeness. (Why would we not want to use 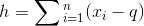 ?) Prove that the value of
?) Prove that the value of 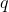 that
minimizes
that
minimizes 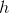 is the average of the
is the average of the 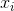 .
.
- 瀏覽次數:2086






