You are here

Home » Brief Calculus » Complex number techniques

Partial fractions revisited

12 November, 2015 - 16:15
Available under Creative Commons-ShareAlike 4.0 International License.

Suppose we want to evaluate the integral
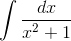
\int \frac{dx}{x^{2}+1}
by the method of partial fractions. The quadratic formula tells us that the roots are iand −i, setting
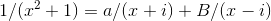
1/(x^{2}+1)=a/(x+i)+B/(x-i)
gives A = i/2 and B = -i/2, so
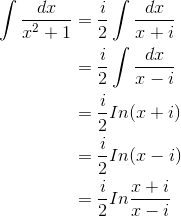
\begin{align*} \int \frac{dx}{x^{2}+1}&=\frac{i}{2}\int \frac{dx}{x+i} \\&=\frac{i}{2}\int \frac{dx}{x-i} \\&=\frac{i}{2}In(x+i) \\&=\frac{i}{2}In(x-i) \\&=\frac{i}{2}In\frac{x+i}{x-i} \end{align*}

The attractive thing about this approach, compared with the method used on page 88, is that it doesn’t require any tricks. If you came across this integral ten years from now, you could pull out your old calculus book, flip through it, and say, “Oh, here we go, there’s a way to integrate one over a polynomial — partial fractions.” On the other hand, it’s odd that we started out trying to evaluate an integral that had nothing but real numbers, and came out with an answer that isn’t even obviously a real number.

But what about that expression (x+i)/(x−i)? Let’s give it a name, w. The numerator and denominator are complex conjugates of one another. Since they have the same magnitude, we must have |w|= 1, i.e., wis a complex number that lies on the unit circle, the kind of complex number that Euler’s formula refers to. The numerator has an argument of 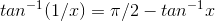tan^{-1}(1/x)=\pi /2-tan^{-1}x , and the denominator has the same argument but with the opposite sign. 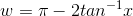Division means subtracting arguments, so arg w=\pi -2tan^{-1}x. That means that the result can be rewritten using Euler’s formula as
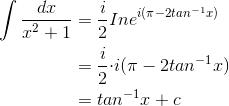
\begin{align*} \int \frac{dx}{x^{2}+1}&=\frac{i}{2}Ine^{i(\pi -2tan^{-1}x)} \\&=\frac{i}{2}{\cdot i(\pi -2tan^{-1}x)} \\&=tan^{-1}x+c \end{align*}                                                
In other words, it’s the same result we found before, but found with- out the need for trickery.

Example 93

Evaluate 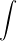\intdx/sin x.

This can be tackled by rewriting the sine function in terms of complex exponentials, changing variables to u= 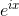e^{ix}, and then using partial fractions.

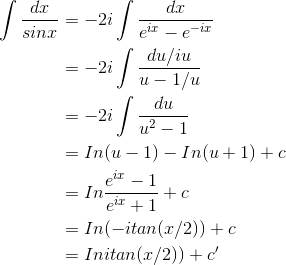
\begin{align*} \int \frac{dx}{sinx}&=-2i\int \frac{dx}{e^{ix}-e^{-ix}} \\&=-2i\int \frac{du/iu}{u-1/u} \\&=-2i\int \frac{du}{u^{2}-1} \\&=In(u-1)-In(u+1) +c \\&=In\frac{e^{ix}-1}{e^{ix}+1}+c \\&=In(-itan(x/2))+c \\&=Initan(x/2))+c{}' \end{align*}                                           

  • 2459 reads