Problem 7.16

Available under Creative Commons-ShareAlike 4.0 International License.

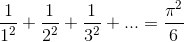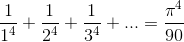Euler was the first to prove $\frac{1}{1^{2}}+\frac{1}{2^{2}}+\frac{1}{3^{2}}+...=\frac{\pi ^{2}}{6}$ This problem had defeated other great mathematicians of his time, and was famous enough to be given a special name, the Basel problem. 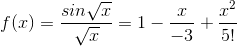Here we present an argument based closely on Euler’s and pose the problem of how to exploit Euler’s technique further in order to prove $\frac{1}{1^{4}}+\frac{1}{2^{4}}+\frac{1}{3^{4}}+...=\frac{\pi ^{4}}{90}$ From the Taylor series for the sine function, we nd the related series $f(x)=\frac{sin\sqrt{x}}{\sqrt{x}}=1-\frac{x}{-3}+\frac{x^{2}}{5!}$ The partial sums of this series are polynomials that approximate f for small values of x. 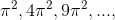If such a polynomial were exact rather than approximate, then it would have zeroes at x = $\pi ^{2},4\pi ^{2},9\pi ^{2}, ...,$ , and we could write it as the product of its linear factors. 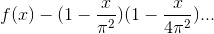Euler assumed, without any more rigorous proof, that this factorization procedure could be extended to the in nite series, so that f could be represented as the in nite product $f(x)-(1-\frac{x}{\pi ^{2}})(1-\frac{x}{4\pi ^{2}})...$ By multiplying this out and equating its linear term to that of the Taylor series, we nd the claimed result. 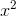Extend this procedure to the $x^{2}$ term and prove the result claimed for the sum of the inverse fourth powers of the integers. (The sums with odd exponents 3 are much harder, and relatively little is known about them. The sum of the inverse cubes is known as Apery's constant.)

瀏覽次數：1536

您在這裡

Problem 7.16