Historically, the calculus of infinitesimals as created by Newton and Leibniz was reinterpreted in the nineteenth century by Cauchy, Bolzano, and Weierstrass in terms of limits. All mathematicians learned both languages, and switched back and forth between them effortlessly, like the lady I overheard in a Southern California supermarket telling her mother, “Let’s get that one, con los nuts.” Those who had been trained in infinitesimals might hear a statement using the language of limits, but translate it mentally into infinitesimals; to them, every statement about limits was really a statement about infinitesimals. To their younger colleagues, trained using limits, every statement about infinitesimals was really to be under- stood as shorthand for a limiting process. When Robinson laid the rigorous foundations for the hyper- real number system in the 1960’s, a common objection was that it was really nothing new, because every statement about infinitesimals was really just a different way of expressing a corresponding statement about limits; of course the same could have been said about Weierstrass’s work of the preceding century! In reality, all practitioners of calculus had realized all along that different approaches worked better for different problems; Problem 4.13 is an example of a result that is much easier to prove with infinitesimals than with limits.
The Weierstrass definition of a limit is this:
Definition of the limit
We say that 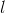 is the limit of the function
is the limit of the function 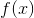 as
as 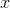 approaches
approaches  , written
, written
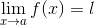
if the following is true: for any real number  , there exists
another real number
, there exists
another real number 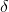 such that for all
such that for all 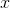 in the interval
in the interval 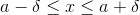 , the value of
, the value of 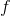 lies within the range from
lies within the range from 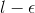 to
to 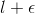 .
.
Intuitively, the idea is that if I want you to make 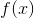 close to
close to
 ,just have to tell you how close, and you can tell me that it
will be that close as long as x is within a certain distance of a.
,just have to tell you how close, and you can tell me that it
will be that close as long as x is within a certain distance of a.
In terms of infinitesimals, we have:
Definition of the limit
We say that  is the limit of the function
is the limit of the function
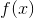 as
as 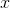 approaches
approaches  , written
, written
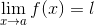
if the following is true: for any infinitesimal number dx, the value of  is finite, and the standard part of
is finite, and the standard part of 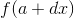 equals
equals 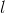 .
.
The two definitions are equivalent. As remarked previously, the derivative 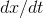 can be defined as the limit
can be defined as the limit 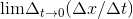 , and if we use the Weierstrass definition of the limit, this means that the derivative
can be defined entirely in terms of the real number sys- tem, without the user of hyperreal numbers.
, and if we use the Weierstrass definition of the limit, this means that the derivative
can be defined entirely in terms of the real number sys- tem, without the user of hyperreal numbers.
Sometimes a limit can be evaluated simply by plugging in numbers:
Example
Evaluate
Plugging in  , we find that the limit is 1.
, we find that the limit is 1.
In some examples, plugging in fails if we try to do it directly, but can be made to work if we massage the expression into a different form:
Example
Evaluate
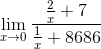
Plugging in 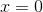 fails because division by zero is undefined.
fails because division by zero is undefined.
Intuitively, however, we expect that the limit will be well defined, and will equal 2, because for very small values of 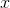 , the numerator is dominated by the
, the numerator is dominated by the 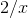 term, and the denominator by the
term, and the denominator by the 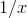 term, so the 7 and 8686 terms will matter less and less as
term, so the 7 and 8686 terms will matter less and less as 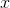 gets smaller and smaller.
gets smaller and smaller.
To demonstrate this more rigorously, a trick that works is to multiply both the top and the bottom by 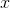 , giving
, giving
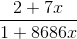
which equals 2 when we plug in 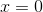 , so we find that the limit is zero.
, so we find that the limit is zero.
This example is a little subtle, because when 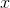 equals zero, the function is not
defined, and moreover it would not be valid to multiply both the top and the bottom by
equals zero, the function is not
defined, and moreover it would not be valid to multiply both the top and the bottom by 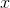 . In general, it’s not valid algebra
to multiply both the top and the bottom of a fraction by 0, because the result is 0/0, which is undefined. But we didn’t actually multiply both the top and the bottom by zero, because we
never let
. In general, it’s not valid algebra
to multiply both the top and the bottom of a fraction by 0, because the result is 0/0, which is undefined. But we didn’t actually multiply both the top and the bottom by zero, because we
never let 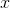 equal zero. Both the Weierstrass
definition and the definition in terms of infinitesimals only refer to the properties of the function in a region very close to the limiting point, not at the limiting point itself.
equal zero. Both the Weierstrass
definition and the definition in terms of infinitesimals only refer to the properties of the function in a region very close to the limiting point, not at the limiting point itself.
This is an example in which the function was not well defined at a certain point, and yet the limit of the function was well defined as we approached that point. In a case like this, where there is only one point missing from the domain of the function, it is natural to extend the definition of the function by filling in the “gap tooth.” Example below shows that this kind of filling- in procedure is not always possible.
Example
Investigate the limiting behavior of 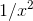 as
as 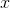 approaches 0, and 1.
approaches 0, and 1.
At 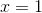 , plugging in works, and we find that the limit is 1.
, plugging in works, and we find that the limit is 1.
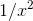
At 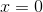 , plugging in doesn’t work, because
division by zero is undefined. Applying the definition in terms of infinitesimals to the limit as
, plugging in doesn’t work, because
division by zero is undefined. Applying the definition in terms of infinitesimals to the limit as 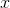 approaches 0, we need to find out whether
approaches 0, we need to find out whether 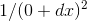 is finite for infinitesimal dx , and if so, whether it al- ways has the same standard part. But clearly
is finite for infinitesimal dx , and if so, whether it al- ways has the same standard part. But clearly 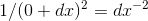 is always infinite, and we conclude that this limit is
undefined.
is always infinite, and we conclude that this limit is
undefined.
Example
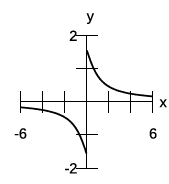
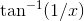
Investigate the limiting behavior of 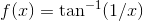 as
as
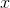 approaches 0.
approaches 0.
Plugging in doesn’t work, because division by zero is undefined.
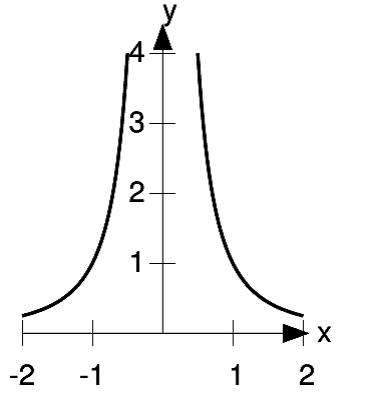
In the definition of the limit in terms of infinitesimals, the first requirement is that 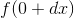 be finite for infinitesimal values of
be finite for infinitesimal values of 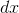 . The graph makes this look plausible, and indeed we can prove that it is true by the transfer principle. For any real
. The graph makes this look plausible, and indeed we can prove that it is true by the transfer principle. For any real
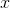 we have
we have  , and by the transfer principle this holds for the hyperreals as well, and therefore
, and by the transfer principle this holds for the hyperreals as well, and therefore
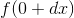 is finite.
is finite.
The second requirement is that the standard part of 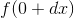 have a uniquely defined value. The
graph shows that we really have two cases to consider, one on the right side of the graph, and one on the left. Intuitively, we expect that the standard part of
have a uniquely defined value. The
graph shows that we really have two cases to consider, one on the right side of the graph, and one on the left. Intuitively, we expect that the standard part of 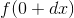 will equal
will equal 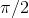 for positive
for positive 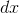 , and
, and 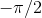 for negative, and thus the second part of the definition will not be satisfied. For a more formal proof, we can use the
transfer principle. For real
for negative, and thus the second part of the definition will not be satisfied. For a more formal proof, we can use the
transfer principle. For real 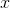 with
with 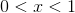 , for example,
, for example, 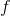 is always positive and greater than 1, so we conclude based on the transfer principle that
is always positive and greater than 1, so we conclude based on the transfer principle that 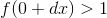 for positive infinitesimal
for positive infinitesimal 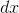 . But on similar grounds we can be
sure that
. But on similar grounds we can be
sure that 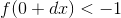 when
when 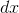 is negative and infinitesimal. Thus
the standard part of
is negative and infinitesimal. Thus
the standard part of 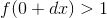 can have different values for
different infinitesimal values of
can have different values for
different infinitesimal values of 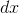 , and we conclude that the limit is
undefined.
, and we conclude that the limit is
undefined.
In examples like this, we can define a kind of one-sided limit, notated like this:
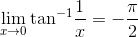
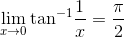
where the notations 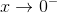 and
and 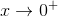 are to be read “as
are to be read “as 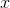 approaches zero from below,” and “as
x approaches zero from above.”
approaches zero from below,” and “as
x approaches zero from above.”
- 2943 reads






