Available under Creative Commons-ShareAlike 4.0 International License.

Figure 2.14
A set of light rays is emitted from the tip of the glamorous movie star’s nose on the film, and reunited to form a spot on the screen which is the image of the same point on his nose. The
distances have been distorted for clarity. The distance y represents the entire length of the theater from front to back.
In a movie theater, the image on the screen is formed by a lens in the projector, and originates from one of the frames on the strip of celluloid film (or, in the newer digital projection
systems, from a liquid crystal chip). Let the distance from the film to the lens be 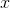 , and let the distance from the lens to the screen be
, and let the distance from the lens to the screen be 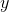 . The projectionist needs to adjust
. The projectionist needs to adjust 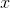 so that it is properly matched with
so that it is properly matched with 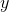 , or else the image will be
out of focus. There is therefore a fixed relationship between
, or else the image will be
out of focus. There is therefore a fixed relationship between 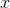 and
and 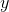 , and this relationship is of the form
, and this relationship is of the form
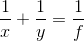
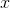 , and let the distance from the lens to the screen be
, and let the distance from the lens to the screen be 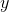 . The projectionist needs to adjust
. The projectionist needs to adjust 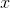 so that it is properly matched with
so that it is properly matched with 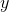 , or else the image will be
out of focus. There is therefore a fixed relationship between
, or else the image will be
out of focus. There is therefore a fixed relationship between 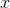 and
and 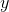 , and this relationship is of the form
, and this relationship is of the form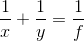
where 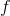 is a property of the lens, called its focal length. A stronger lens has a shorter focal length. Since the theater is large, and
the projector is relatively small,
is a property of the lens, called its focal length. A stronger lens has a shorter focal length. Since the theater is large, and
the projector is relatively small, 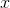 is much less than
is much less than 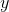 . We can see from the equation that if
. We can see from the equation that if 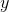 is sufficiently
large, the left-hand side of the equation is dominated by the
is sufficiently
large, the left-hand side of the equation is dominated by the 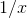 term, and we have
term, and we have 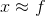 . Since the
. Since the 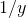 term doesn't completely vanish, we must have
term doesn't completely vanish, we must have 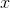 slightly greater than
slightly greater than 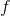 , so that
the
, so that
the 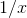 term is slightly less than
term is slightly less than 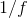 . Let
. Let  , and approximate
, and approximate 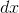 as being infinitesimally small. Find a simple expression for
as being infinitesimally small. Find a simple expression for 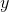 in terms
of
in terms
of 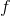 and
and 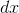 .
.
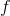 is a property of the lens, called its focal length. A stronger lens has a shorter focal length. Since the theater is large, and
the projector is relatively small,
is a property of the lens, called its focal length. A stronger lens has a shorter focal length. Since the theater is large, and
the projector is relatively small, 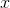 is much less than
is much less than 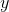 . We can see from the equation that if
. We can see from the equation that if 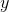 is sufficiently
large, the left-hand side of the equation is dominated by the
is sufficiently
large, the left-hand side of the equation is dominated by the 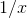 term, and we have
term, and we have 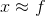 . Since the
. Since the 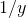 term doesn't completely vanish, we must have
term doesn't completely vanish, we must have 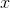 slightly greater than
slightly greater than 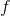 , so that
the
, so that
the 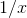 term is slightly less than
term is slightly less than 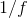 . Let
. Let  , and approximate
, and approximate 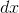 as being infinitesimally small. Find a simple expression for
as being infinitesimally small. Find a simple expression for 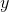 in terms
of
in terms
of 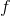 and
and 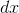 .
.
- 2421 reads






